

Bouncing Barney
by
Ana Kuzle
Problem:
Barney is in the triangular room shown here. He walks from a point on BC parallel to AC. When he reaches AB, he turns and walks parallel to BC. When he reaches AC, he turns and walks parallel to AB. Prove that Barney will eventually return to his starting point. How many times will Barney reach a wall before returning to his starting point? Explore and discuss for various starting points on line BC, including points exterior to segment BC. Discuss and prove any mathematical conjectures you find in the situation.
If we look at the GSP file, we can see that we can observe several situations. We can also observe Barney's walk in steps that will be used for our explorations. From the file we can see that the path has a finite existence as well. Thus, we will focus this exploration by proving that his path is finite, and look at several situations depending on the initial point of Barney's start. Also, by measuring will try to see whether the total length Barney walks is invariant of the perimeter of the triangle ABC or not.
I. The starting point is on the segment BC
a) the starting point D is an arbitrary point on the segment BC
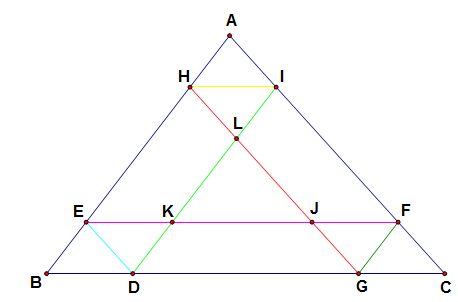 |
<EBD=<FGC=<AHI because they are alternate interior angles. For the same reason, <BDE=<GCF=<HIA. Thus, <BED=<GFC=<HAI. Since we know that BGFE is a parallelogram from the construction, BE=GF. According to ASA postulate, triangles BDE, GCF are congruent. Similarly, since BDIH is a parallelogram, BD=HI. Thus, triangles BDE, and HIA are congruent. Thus, triangles BDE, GCF, and HIA are congruent. Let us take a closer look on a triangle ABC. Triangle ABC, and BDE share a same angle at a vertex B, <BDE=<BCA (alternate angles), thus according to AA postulate triangle ABC is similar to triangle BDE, GCF, and HIA. Let denote BD=GC=HI=x, DE=CF=IA=y, and GF=BE=HA=z. Naturally, AB=c, BC=a, and AC=b. Also, DG=a-2x, FI=b-2y, and EH=c-2z. Thus, l=2x+a-2x+2y+b-2y+2z+c-2z=a+b+c=o(ABC). Therefore, the total distance that Barney walks is equal to the perimeter of the triangle ABC. Having in mind that we assumed the path was finite to find the distance, let prove that the path has a finite existence, In order to prove that, we can give an algebraic proof, or a more elegant one, geometric. Geometric proof. DCEF is a parallelogram. Thus, DE=CF. Since <B=<G, and <D=<C, triangles BDE, and GCF are congruent. Also, GCIH is a parallelogram. Thus, GC=HI. But, BD=GC, so BD=HI. Furthermore, MBIH is a parallelogram (M being the point on the segment BC after reaching the wall 5 time). Thus, HI=BM. But HI=BM, so BD=BM. Therefore D=M, and we can conclude that Barney's path has a finite existence. As said before, looking at Barney's walk step by step, we can see that Barney reaches the wall 5 times before returning to the initial point. |
b) The starting point is at the vertex B.
This is a special case of case a). We can ask ourselves the number of cycles Barney travels? Since we know Barney bounces every time he reaches the wall, he makes 2 cycles each time half of the length of the sides. Therefore, the length of the walk is equal to the perimeter of the triangle ABC.
c) The starting point is equal to the midpoint of the segment BC.
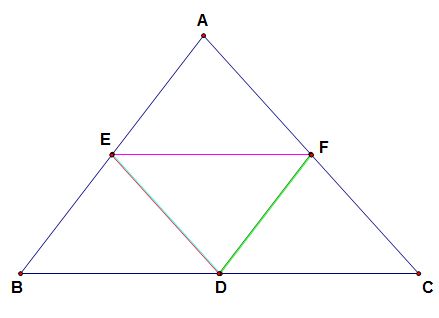 |
Using the properties of the midpoint, and parallel lines we can conclude that the triangles BDE, DCF, EFA, and FED are congruent, and similar to the triangle ABC. Barney returns to the initial point after he reaches the wall 2 times. Since the midsegement is equal to half of the length of the base, we can conclude that the total distance that Barney walks is equal to half of the perimeter of the triangle ABC. |
d) The starting point is at either of two points on the segment BC that divides BC into three equal parts.
 |
Having this situation, we get 9 congruent triangles that are similar to the triangle ABC. Again, this is true using properties of parallel lines. Also, the three inner triangles coincide at one point. That point is the centroid of the triangle ABC. Barney reaches the wall 5 times, and he passes the centroid 3 times before returning to the starting point. What is the length of his path? Again we have that l=y+2x+z+2y+x+2z=3x+3y+3z. But, we know that points D, E, F, G, H, and I divide sides of the triangle ABC into three equal parts so we ca conclude that 3x=a, 3y=b, and 3z=c. Thus, the total distance Barney walks is equal to the perimeter of the triangle ABC. |
II. The starting point is inside the triangle ABC.
a) the starting point is an arbitrary point inside the triangle ABC
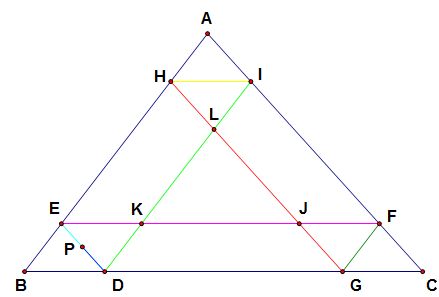 |
Let P be an arbitrary point inside the triangle ABC. If we construct a parallel to AC through P, it intersects BC, and AB at D, and E respectively. Thus, we have the situation from I.a). Using the same arguments as before, the total length of Barney's walk is equal to the perimeter of the triangle ABC. Since Barney starts walking from a point inside the triangle, he reaches the wall 6 times before he returns back to the starting point P. |
b) the starting point is the centroid of the triangle ABC
Now, we can refer to I.d) and II. s). We have the same conclusions about the length of Barney's walk being the same as the perimeter of the triangle ABC, and dividing the triangle ABC into 9 congruent triangles similar to the triangle ABC. Also, Barney walks 2 times through the centroid and reaches the wall 6 times before returning back to it.
c) the starting point is the orhocenter of the triangle ABC
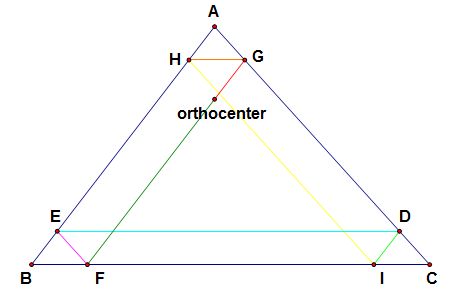 |
Orhocenter is the starting point of Barney's walk. Again the parallel to AB through the orthocenter intersects AC, and BC to points G, and F respectively. Also, triangles BFE, ICD, and HGA are congruent for the same reasons as before. Hence, the path has a finite existence. If we look at the distance Barney walks, again using the same reasons as before we can conclude that his distance is equal to the perimeter of the triangle ABC. Furthermore, Barney reaches the wall 6 times before returning to the initial point, the orthocenter. In his walk, he does not pass through the orthocenter as in the case with the centroid. |
III. The initial point is outside the triangle ABC
a) the initial point is outside the segment BC, but on the line BC
 / / |
Let D be on the extension of the line BC. Also, let us extend all of the sides of the triangle ABC. Thus we get that, E is the intersection of a parallel to AB through D with AC. F is the intersection of a parallel to BC through E with AB. G is the intersection of a parallel to AC through F with BC. H is the intersection of a parallel to AB through G with AC. I is the intersection of a parallel to BC through H with AB. Furthermore, triangles ABC, AFE, IBD, and HGC are similar to the triangle ABC. Thus since the triangles mentioned above are similar, Barney returns to the point D, the initial point, after reaching the wall 5 times. It is obvious from the picture that the total distance of Barney's walk is greater than the perimeter the triangle ABC. Let us prove that. We are going to find the total distance Barney walks actually. |
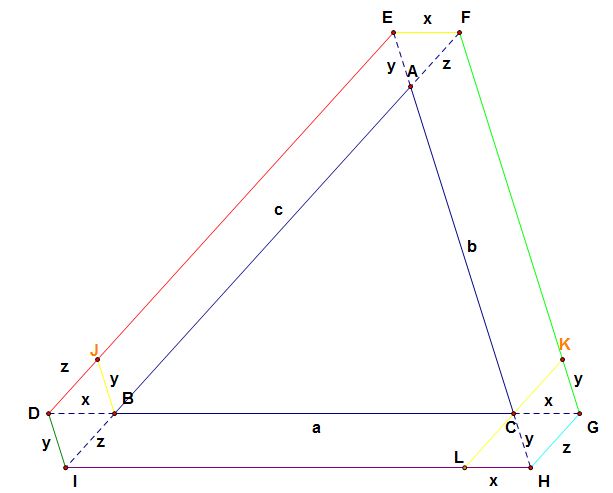 |
The length of the walk is equal to: l=DE+EF+FG+GH+HI+ID. But DE=DJ+JE, and BAEJ is a parallelogram. Thus, AB=JE=a. Similarly, AFKC is a parallelogram. Thus, AC=FK=b. Again, since ILCB is a parallelogram we have that BC=IL=a. Also, the triangles DBJ, FEA, CGK, and LHC are similar (properties of parallel lines). Thus we get: l=DJ+JE+EF+FK+KG+GH+HI+ID. Furthermore, l=z+c+x+b+y+z+x+a+y=a+b+c+2(x+y+z). Thus, l=o(ABC)+2o(AFE). We have proven that the distance Barney walks is equal to the sum of the perimeter of the triangle ABC and twice the sum of x, y, and z. |
Click here to see the GSP file.
b) the initial point is an arbitrary point outside the triangle ABC
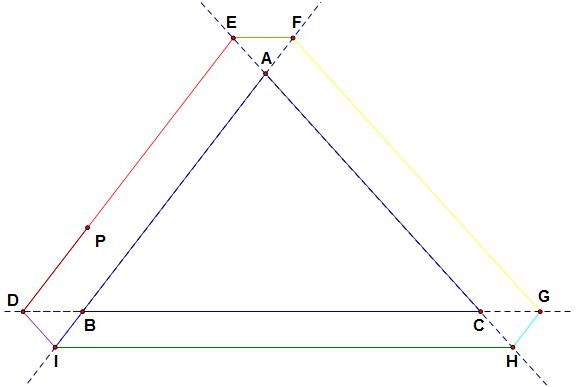 |
Point P is an arbitrary point outside the triangle ABC. We can refer here to III. a). Triangles DBI, EFA, and CGH are congruent and similar to the triangle ABC. Thus, the path has a finite existence. The path that Barney walks is the same as the one from III. a). Hence, the distance of the walk is equal to the perimeter of the triangle ABC. Furthermore, Barney reaches the wall 6 times before returning to the initial point P. |
Conclusions:
Barney returns to the starting point regardless where he starts his walk.
If Barney starts from an arbitrary point on the line BC, he returns back after reaching the wall 5 times.
If Barney starts from a midpoint on the segment BC, he returns back after reaching the wall 2 times.
If Barney starts from an arbitrary point inside the triangle ABC, he returns back after reaching the wall 6 times.
If Barney starts from a centroid or an orthocenter, he returns back after he reaches the wall 6 times.
If Barney starts his walk from an arbitary point outside the triangle, he returns back after he reaches the wall 6 times.
The total distance Barney walks is equal to half of the perimeter of the triangle ABC if he starts from a midpoint on BC.
If he starts from a point outise the triangle ABC, then the total distance he walks is equal to the sum of the perimeter of the triangle ABC and twice the sum of x, y, and z.
In all other cases explored here, the length he walks is equal to the perimeter of the triangle ABC.
Hence, the total length Barney walks in not an invariant of the perimeter of the triangle ABC. Also, with no loss of generality all conclusions hold regardless of a starting point on segment/line.
Extension of the problem.
It would be interesting to see what happens if Barney is places in a hexagonal room. Click here to observe some of the possible situations.
I will just look at one of the cases. Either way, other cases are analogous to a case when Barney was placed in a triangular room.
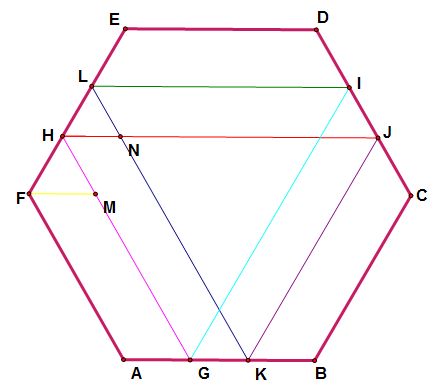 |
Barney starts his walk from point G on a segment AB. It makes a walk GH, HJ, JK, KL, LI, and IG. Note that segments FM, and HN are here to help us for showing the length of the distance he walks. Barney reaches the wall 5 times before returning to the starting point. Furthermore, the distance he walks is a obviously greater than the perimeter of the hexagon. The question is how much. Without going too much into detail but knowing that we are again using the properties of parallel lines and equilateral triangle we have that the distance Barney walks is equal to the sum of the perimeter of the hexagon ABCDEF, twice the sum of the perimeter of the triangle FMH, and perimeter of the triangle HNL. |